It rewards the quality of a research work in mathematics and its applications, which particularly stands out for its originality, the depth and rigor of its purpose, the scope of the results obtained and their applicability or publishability. Particular importance is attached to the candidates' personality, maturity, charisma, personal involvement in scientific work and ability to present results clearly and appropriately to a non-specialist audience.

Curriculum Vitae of Frank Callier.

Title: Modeling dynamical systems using latent differential equations

Promoter : Annick Sartenaer

Co-promoter : Noémie Vlaminck (Cenaero)

Supervisor : Cédric Simal

Summary:

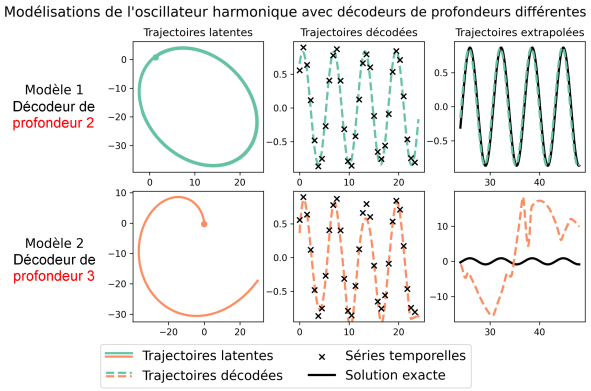
Time series modeling is a recurring problem in many fields such as industrial optimization or epidemiology. In this work carried out in collaboration with Cenaero and Carmeuse Technologies, we study the ability of the latent differential equation (LDE) model to reconstruct a dynamic system based on time series. The aim is to provide a coherent model that respects certain properties, namely those of dynamic systems. EDL combines a neural differential equation, delivering the expected "dynamic" coherence, with elements from generative models, delivering the flexibility often required to address the modeling problem. If the EDL can achieve a suitable modeling result, it nevertheless appears to be sensitive to the respective strengths of its two components. A generative component that is too powerful could take precedence over the dynamic component, thereby undermining the model's coherence, while too little power could totally hinder its operation. It follows that the EDL should be improved in this respect, by constraining the dynamic component without directly limiting its power. One solution could be the addition of a regularization term to the model's entraınement criterion, in order to lead to the conservation of topological properties within it.